Problems

In Problems 1-12, find the area of the surface generated by rotating the given curve about the y-axis.

first quadrant

In Problems 13-20, find the area of the surface generated by rotating the given curve about the x-axis.

21             The part of the circle x² + y² = r² between x = 0 and x = a in the first quadrant is rotated about the x-axis. Find the area of the resulting zone of the sphere (0 < a < r).

22            Solve the above problem when the rotation is about the y-axis.

In Problems 23-26 set up integrals for the areas generated by rotating the given curve about (a) the y^:-axis, (b) the x-axis.

27            Set up an integral for the area generated by rotating the curve y = jx², 0 < x < 1 about the x-axis and find the Trapezoidal Approximation with Δx = 0.2.

28            Set up an integral for the area generated by rotating the curve y = jx³, 0 < x < 1 about the y-axis and find the Trapezoidal Approximation with Δx = 0.2.

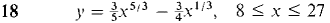
29            Show that the surface area of the torus generated by rotating the circle of radius r and center (c, 0) about the y-axis (r < c) is A = 4π²rc. Hint: Take y as the independent variable and use the formula for the length of the arc of the circle from y = a to y = b.