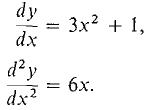| The ebook Elementary Calculus is based on material originally written by H.J. Keisler. For more information please read the copyright pages. |

|

Home  Continuous Functions Continuous Functions  Derivatives and Curve Sketching Derivatives and Curve Sketching  Examples Examples  Example 1 (Continued): Change of Direction Example 1 (Continued): Change of Direction |
|||||||||||||||||






|
|||||||||||||||||
Example 1 (Continued): Change of Direction
y = x3 + x - 1. We have
dy/dx is always positive, while d2y/dx2 = 0 at x = 0. We make a table of values for y and its first two derivatives at x = 0 and at a point to the right and left side of 0. With the aid of Theorems 1-3, we can draw the following conclusions:
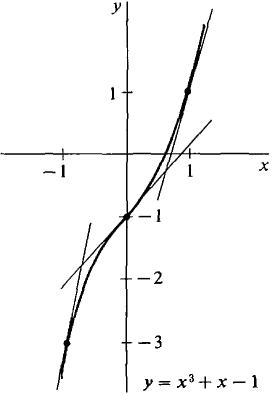
At the point x = 0, the curve changes from concave downward to concave upward. This is called a point of inflection.
To sketch the curve we first plot the three values of y shown in the table, then sketch the slope at these points as shown in Figure 3.7.7, then fill in a smooth curve, which is concave downward or upward as required.
Figure 3.7.7
|
|||||||||||||||||
Home  Continuous Functions Continuous Functions  Derivatives and Curve Sketching Derivatives and Curve Sketching  Examples Examples  Example 1 (Continued): Change of Direction Example 1 (Continued): Change of Direction |
|||||||||||||||||
Last Update: 2006-11-15