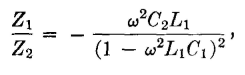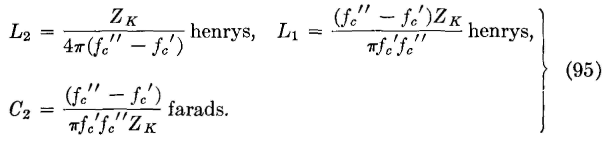The Band-Elimination Filter

Figure 39. Attenuation curve for three constant-k band-pass filter sections such as Fig. 38. Terminated in 600 ohms resistance.

and

since, for the constant-k filter, L₁C₁ = L₂C₂- A filter of this type will pass all frequencies such that Z₁/Z₂ will lie between 0 and -4. Thus,

the solution of which is

Figure 40. Reactance curves for a non-confluent double band-pass filter, passing the two bands T and T'. This figure is not for the filter of Figs. 38 and 39.

Figure 41. A band-elimination filter of the constant-k type. Two cutoff frequencies are obtained,

and

To find the upper and lower frequency limits, the impedance ratio is equated to zero. Thus, Z₁/Z₂ = -ω_c²C₂L₁/(1 - ω_c²L₁C₁) = 0, and from this, two values ω_c = 0 and ω_c = ∞ are obtained. From these two relations and from the preceding paragraph it follows that the band-elimination filter will pass all frequencies from zero to f_c' cycles per second, will attenuate all frequencies from f_c' to f_c", and will pass all currents having frequencies between f_c" and infinity. This filter then eliminates the band f_c' to f_c".

The method of finding the design equations is similar to that used for the band-pass filter. If the two expressions of equation 93 are subtracted, it will be found that (f_c" - f_c') = 1/(4πsqrt(L₂C₁)). Since, for the constant-k filter, L₁C₁ = L₂C₂ and Z_K = sqrt(L₂/C₁) = sqrt(L₁/C₂). this equation can be solved for C₁, and

Infinite and zero values occur at

Using

these relations and the equations just obtained, it can easily be shown that

Calculations for the various inductors and capacitors for a simple band-elimination filter are made from equations 94 and 95.