Current Gain

Author: Leonard Krugman

In view of these limitations, the basic circuit illustrated in Fig. 7-1 is not a useful approximation of transistor performance at high frequencies. To modify this circuit for accurate representation of high frequency equivalence requires that all of the internal parameters be specified in a complex form (magnitude and phase angle) as functions of the frequency. In most cases, however, it is sufficiently accurate to modify Fig. 7-1 to include only the variation of α with frequency, since few design problems justify the details required for exact equivalence. The variation in current gain can be satisfactorily approximated by the relationship:

where α is the current gain of the operating frequency f; α₁ is the low frequency current gain; and f_c is the frequency at which the current gain is 0.707 of its low frequency value (3 db down).

As a numerical example of the above, compute the current gain tor a junction transistor having a low frequency current gain of _ai = 0.95, an _a cut-off frequency of f_c = 10 me, and an operating frequency of 7.5 me. Then

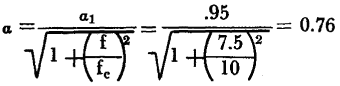
Including only the junction capacitance and variation in _a in the low frequency circuit makes all the computed values far from exact. In addition to the capacitive reactance of the emitter, there is also considerable variation with frequency in the collector resistance and collector junction capacitance. The collector resistance r_c decreases rapidly for a ratio of greater than 0.15, falling to about 10% of its low frequency value at = 1, and then remains at that value. The collector junction capacitance C_c also decreases as the operating frequency increases above an greater than 0.15, but does not decrease as rapidly as r_c. In a typical characteristic, C_c drops to approximately 75% of its low frequency value a =1 and to about 50% at=10, after which the curve levels out.Due to the coupling betweenthe input and output circuits, , the input impedance contains a reactive componentbeyond the emitter shunt capacitance. At the α cut-off frequency f_c, the reactive component is approximately equal to the resistive input component. This causes the input impedance to be inductive for the grounded base connection, and capacitive for the grounded emitter connection (due to phase reversal).