| Transistor Basics is a free introductory textbook on transistors and their basic applications. See the editorial for more information.... |

|

Home  The Grounded Base Transistor The Grounded Base Transistor  The Grounded Base Connection The Grounded Base Connection  Negative Resistance Negative Resistance |
||||||||||
| See also: Conditions for Oscillation, Basic Types | ||||||||||






|
||||||||||
|
Negative Resistance and Transistor StabilityAuthor: Leonard Krugman Consider the general expression for input resistance
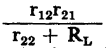 . This condition is most difficult to attain when the output is shorted, namely when RL = 0. For the transistor to be stable under this condition, r11r22 must be greater than r12r21. The stability factor is the ratio of r12r21 to r11r22. The stability factor . This condition is most difficult to attain when the output is shorted, namely when RL = 0. For the transistor to be stable under this condition, r11r22 must be greater than r12r21. The stability factor is the ratio of r12r21 to r11r22. The stability factor  must be less than unity for short-circuit stability. Substituting the equivalent transistor parameters for the grounded base connection into the stability equation, the following relationship is obtained: must be less than unity for short-circuit stability. Substituting the equivalent transistor parameters for the grounded base connection into the stability equation, the following relationship is obtained:
Expanding equation 3-42, rcre + rcrb + rbre + rb2 > rhrm + rb2 Dividing through by rb
This equation emphasizes the importance of the backward transfer resistance rb, since when rb = 0, the transistor must have a positive input resistance. On the other hand, if the value of rb is increased by adding external resistance, it is possible to reach a condition where a normally positive input resistance becomes negative. Notice, however, that increasing the total base resistance eventually causes the input resistance to become negative only if re + rc is less than rm. In the case of the junction transistor, rc is always greater than rm, and increasing the base resistance cannot produce a negative input resistance. The conditions for negative output resistance are obtained similarly. In the general output resistance equation,
the output resistance ro is positive provided that r22 is greater than
This condition for stability is most difficult to meet when the generator resistance is equal to zero. For the transistor to be stable under this condition, rnr22 again must be greater than r12r21. The same stability factor and equations then exist for both the input and output resistances. It is evident, then, that one method of fabricating a transistor oscillator is by adding sufficient resistance to the base arm. Typical circuits incorporating this principle will be considered in Chapter 6.
|
||||||||||
Home  The Grounded Base Transistor The Grounded Base Transistor  The Grounded Base Connection The Grounded Base Connection  Negative Resistance Negative Resistance |
||||||||||
Last Update: 2010-11-17