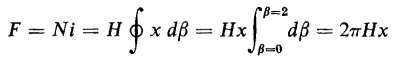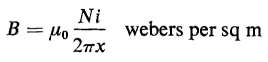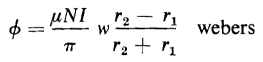| Capacitors, Magnetic Circuits, and Transformers is a free introductory textbook on the physics of capacitors, coils, and transformers. See the editorial for more information.... |

|

Home  Magnetic Circuits Magnetic Circuits  The Toroid The Toroid |
|||||||||||||||||||||||






|
|||||||||||||||||||||||
The Toroidal Coil
Figure 3-9(a) shows a toroidal coil with a uniformly distributed winding of N turns carrying a practically constant current of i amp. A toroid may be shaped like a doughnut or it may be in the form of a hollow cylinder such as is shown in Fig. 3-9(a). If the number of turns N is large, the current will produce magnetic lines of flux that are concentric circles confined to the toroid. This is evident from the direction of the flux lines through the plane of a rectangular loop carrying current as illustrated in Fig. 3-9(b). The magnetic flux is in a direction normal to that plane.
Each turn in the winding of Fig. 3-9(a) is such a loop, and if the number of turns or loops is large the flux will be normal to the radius everywhere in the toroid. This means that the flux lines will be concentric circles. Consider the elemental flux path of thickness dx and radius x in the toroid. The width of this elemental path is the width of the toroid, namely w. Since this path closed upon itself, being a circle, Ampere's circuital law can be applied to express the magnetic field intensity in terms of the radius x. All the turns in the winding link the flux path, hence the total current that links the elemental path is the product of the current / and the number of turns N. Therefore, according to Eq. 3-34, we have
From Fig. 3-9(a) it is apparent that in the scalar product H
Since the flux lines are concentric circles and if the material in the toroid has constant magnetic permeability, as is the case for free space, air and most nonferrous materials, H is constant everywhere in the circular path of radius x. Under these conditions the magnetic field intensity H in the line integral of Eq. 3-35 is a constant multiplier when coupled with the relationship expressed in Eq. 3-36. As a result Eq. 3-35 can be reduced as follows for this simple circular path
where F the magnetomotive force or mmf expressed in ampere turns. Then from Eq. 3-37 we get
Substitution of Eq. 3-15 in Eq. 3-38 yields the expression for the magnetic flux density in the circular path of radius x as follows
The magnetic flux crossing the incremental area dA = w dx in Fig. 3-9(a) is expressed by
where Θ is the angle between the flux density vector B and the normal to the vector dA. Since the vector associated with areas is perpendicular to the surface of the area being represented, the angle Θ in this case is zero. The vector B is tangent to the circle and the surface of the area w dx is radial. Then from Eqs. 3-39 and 3-40 we get
The total flux within the toroid is found by integrating as follows
Materials known as magnetic materials are ferrous materials and certain alloys of metals. They have a magnetic permeability that is much greater than that of free space. In the rationalized MKS system of units the permeability μ is taken as the product μrμ0 where μ0 = 4μ x 10-7 h per m, the magnetic permeability of free space and μr = the relative permeability of the toroid. The relative permeability of ferromagnetic materials varies not only with the kind of material but also varies with the flux density in a given material. For example 24 gauge U.S.S. Electrical Sheet Steel has a relative permeability of about 1,300 at a value of H of about 24 amp turns per m, rising to a maximum of 5,800 at a value of H of about 120 amp turns, then decreasing to 1,300 at 960 amp turns per m. If the toroid consists of a magnetic material that has a uniform relative permeability μr the total flux in the toroid is expressed by
In many applications it is sufficient to take H as the total ampere turns divided by the mean length of flux path. The value of B, resulting therefrom, multiplied by the area normal to the mean path is assumed to give the total flux. Thus for the toroid of Fig. 3-9(a) this approximation yields
and A = (r2 - r1)w, hence
In general, if the magnetic circuit has a uniform cross-sectional area A normal to the direction of the magnetic flux and if the mean length of the flux path is l, the steady flux or slowly varying flux can be expressed approximately as follows
|
|||||||||||||||||||||||
Home  Magnetic Circuits Magnetic Circuits  The Toroid The Toroid |
|||||||||||||||||||||||
Last Update: 2011-08-01



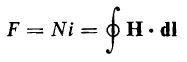
 dl = H cosΘ dl, the product cosΘ dl is the projection of dl on the circle of radius x and is expressed by
dl = H cosΘ dl, the product cosΘ dl is the projection of dl on the circle of radius x and is expressed by