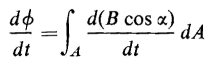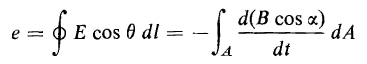Induced EMFs

For its operation, the transformer depends on the emfs that are induced in its various circuits by a common magnetic flux. According to Faraday's Law, the emf induced in a fixed circuit is proportional to the time rate of change of the magnetic flux. If there are N turns of wire in series, through all of which the flux undergoes the same rate of change, the induced emf is

[6-1]

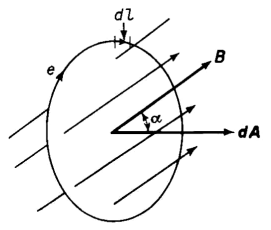
The minus sign is in accordance with Lenz's Law and shows that the induced emf e would produce a current in such a direction as to oppose any change in the flux Φ through the circuit. It is important to note that the opposition is to the change in the flux and not to the flux itself. The sense of the induced emf e in relation to an increasing flux through a closed path is shown in Fig. 6-1.

Figure 6-1. Direction of induced emf around a fixed closed path when the flux through the surface bounded by the path is increasing

The vector dA in Fig. 6-1 represents an elemental area through which the flux density is represented by the vector B at the angle α to the normal of the surface associated with the area vector dA. If E is the magnitude of the electric field intensity due to the varying magnetic flux, then the emf around the closed path is

[6-2]

where E cos Θ is the component of the electric field intensity parallel to the path at a given point. This component of E has the same sense as e. If the integration in Eq. 6-2 is taken around the complete path once, the result is the emf per turn. This emf, however, exists whether the path is occupied by a conducting material or by free space or some other nonconducting material. Hence, we have from Eq. 6-1

[6-3]

as the volts per turn.

The flux Φ which links the area A enclosed by the path in Fig. 6-1, is obtained by integrating the normal component of the flux density B over the area A so that

[6-4]

and

[6-5]

If the configuration of the path is fixed, the area A is constant, and only the quantity B cos α is free to change with time, thus permitting differentiation with respect to time under the integral, and

[6-6]

From Eqs. 6-1, 6-2, and 6-6 it follows that

[6-7]

which can be expressed in vector notation as

[6-8]