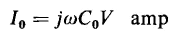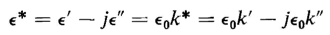Complex Dielectric Constant

The phasor diagram of Fig. 2-25(c) shows the current in an ideal capacitor to lead the applied a-c voltage by an angle of 90°. The dielectric of an ideal capacitor is free space that has a relative dielectric constant of unity and is free of polarization and leakage. If the applied voltage and frequency are kept constant and a solid dielectric or a liquid dielectric, or a combination of both, is made to displace the free space the current will increase because of polarization. In addition the current will now lead the voltage by an angle less than 90° as shown in the phasor diagram of Fig. 2-25(d). For a given applied voltage and frequency, the relative dielectric constant of an imperfect dielectric is the ratio of the current phasor to that which would result by replacing the imperfect dielectric with free space. Because of the dielectric energy loss the relative dielectric constant is a complex quantity rather than a real number.

Let

C₀ = capacitance of the capacitor when it has free space for its dielectric
V = applied sinusoidal voltage
ω = 2πf angular velocity in radians per second
f = frequency in cycles per second
I₀ = current in the capacitor when dielectric is free space
C = apparent capacitance when the dielectric is imperfect
I = current in the capacitor when the dielectric is imperfect

From Fig. 2-25(c)

[2-76]

From Fig. 2-25(e)

[2-77]

the dielectric constant is found by dividing Eq. 2-77 by Eq. 2-76. This yields

[2-78]

The term expressed by Eq. 2-78 is also called the complex relative permittivity of the dielectric in which k" is the relative loss factor.

Another term that is used is the complex permittivity. This is synonymous with absolute dielectric constant and is expressed as

[2-79]

where ε₀ is the dielectric constant of free space, i.e., 8.854 x 10^-12 farads per m.

The dissipation factor DF or loss tangent becomes

[2-80]

Typical values of relative dielectric constant and dissipation factor are shown in Table 2-4.

Table 2-4 shows that the dielectric constant decreases with frequency. In the case of porcelain and Pyranol, the decrease is more pronounced than for Teflon and cable oil. This decrease in the dielectric constant is caused by the inability of some of the larger polar molecules to follow the reversals of the electric field. The dissipation factor tan δ is quite small for all the four materials listed. In the case of liquid dielectrics appreciably larger values of dissipation factor are indications of deterioration or contamination of the dielectrics. In fact, dissipation factor or power factor tests have been used for years to determine the quality of capacitors, high-voltage cable, and dielectric materials themselves.