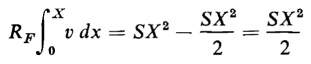Viscous Friction and Spring

Figure 1-9. Mechanical system involving elasticity and friction

Consider a spring that has negligible mass and negligible losses. Suppose it is extended by a force applied through a rope sliding on a surface having a viscous friction such that the force required to overcome the friction is proportional to the velocity at which the rope slides across the surface. Assume the applied force F to be constant. In Fig. 1-9 let

S = coefficient of stiffness of the spring in newtons per m
R_F = friction constant in newtons per meter per second
x = extension of the spring in meters

then

[1-41]

where dx/dt is the velocity v with which the spring is extended, hence

[1-42]

The power is expressed by

[1-43]

The power expressed by the term R_Fv² is converted into heat and the power expressed by the term Svx is the power that stores energy in the spring.

The energy in this system is obtained by taking the time integral of Eq. 1-43 as follows

[1-44]

If x = 0 when t = 0 there results

[1-45]

NOTE: dx = v dt

The stored energy is Sx²/2 and the converted energy is

To determine the relationship between the stored energy and that converted into heat, consider the total energy input for a final extension of the spring to a value X, then

[1-46]

However, the final value of x, namely X, is determined from the relationship

[1-47]

and

[1-48]

A comparison of Eq. 1-48 with 1-46 shows that the energy converted into heat is exactly equal to the energy stored in the spring, in that

[1-49]

This means that when a constant force is applied to such a system, it is capable of storing only one-half the applied energy. This is true regardless of the value of the friction constant R_F. If the parameter R_F is made low, the velocity v goes up correspondingly in such a manner that the frictional energy loss remains constant.

A similar situation exists for an electrical R-C circuit.