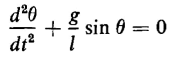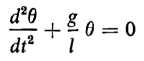The Law of Degradation of Energy

This law, also known as the Second Law of Thermodynamics, governs the irreversible process. It states that differences in energy levels always tend to disappear. Thus, water flows spontaneously from a higher level to a lower; the same is true for heat, which flows from the higher to the lower temperature. The same relationship is valid for the flow of electric charge, because it flows from a higher to lower potential. Equation 1-2 can be expanded into the following form

[1-3]

Consider a stationary electric motor as a system and assume this motor to be started while connected to its load. All of the energy that enters the motor is electrical as long as the ambient temperature does not exceed the temperature of the motor. The two major components of energy that leave the motor are

• The energy supplied to the connected load.
• The heat that escapes to surrounding space.

In addition, there is the energy of vibration and noise, or acoustic energy. The gain in reversible energy would be in the forms of

• Kinetic energy of rotation.
• Kinetic energy stored in the magnetic field.
• Potential energy stored in the electrostatic field (in the insulation).

The gain in irreversible energy in the motor would be that which raises the temperature of the motor, i.e., heat resulting from electrical and magnetic losses as well as from friction and windage losses.

In some systems part or all of the stored reversible energy may change its form from potential to kinetic energy and vice versa. The simple pendulum affords a good example of the periodic change of stored energy. Suppose that a small body C of mass M is suspended by a thread of negligible mass and of length l from point 0 as shown in Fig. 1-3.

Consider the mass originally at rest with the position of the body at A. Assume further that this pendulum is enclosed in a completely evacuated boundary. At rest the body possesses a certain amount of potential energy with reference to some level in space at a distance S below A. If the body C is now displaced to the left through an angle θ₀, to position B, then the energy that entered the system is the increase in potential energy and is expressed by

[1-4]

where g = 9.807 mps², the acceleration of gravity.

If the body is now released and allowed to swing freely, and if there is no friction, then by the time C has traveled from its initial position at the angle θ₀ to the position corresponding to the angle θ it has given up some of its potential energy. This reduction in potential energy must be compensated by an equal amount of energy in some other form.

Figure 1-3. Simple pendulum

In this ease kinetic energy associated with the velocity of the pendulum provides the compensation. That part of the energy put into the system, which is in the form of potential energy when the angle has decreased from θ₀ to θ, is expressed by

[1-5]

and the kinetic energy is

[1-6]

It is evident that when angle θ is zero the body C is at its lowest level. This is also the original level before energy was added. At that instant the potential energy is gmS and the kinetic energy has its maximum value being now equal to W_in. However, as the mass C rises from positions to the right of A, it starts to regain its potential energy and its kinetic energy is reduced. By the time the angle θ has reached a value θ₀ to the right of center, the potential energy is again equal to Win and the oscillation starts toward the left. In the absence of friction and viscosity the pendulum will again reach its original position. This is a reversible process. The period of the pendulum can be determined on the basis of the Law of Conservation of Energy as follows

[1-7]

When Eq. 1-7 is differentiated with respect to time, the result is

[1-8]

but

[1-9]

Equation 1-8 divided by Eq. 1-9 yields

[1-10]

The time derivative of Eq. 1-9 results in

[1-11]

Equation 1-11 substituted in Eq. 1-10 yields

[1-12]

The solution of Eq. 1-12 is not elementary; it involves an elliptic integral. However, if θ is small, then

[1-13]

and Eq. 1-12 can be approximated as follows

[1-14]

It can be shown that the solution of Eq. 1-14 is

[1-15]

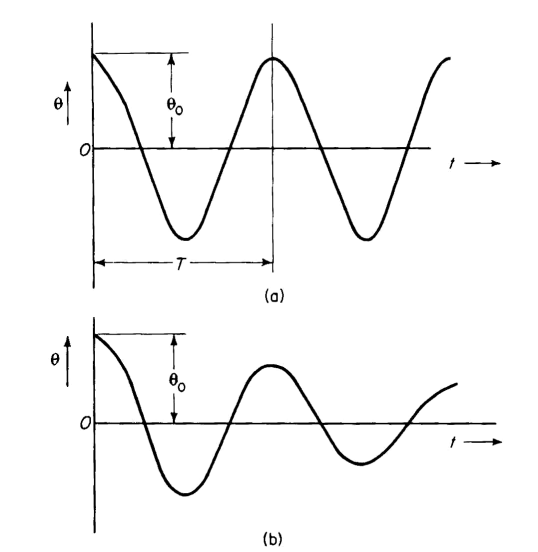
The period T of a pendulum is the time in seconds for one complete oscillation as shown in Fig. 1-4(a). During the interval T the angle in Eq. 1-15 has a value of 2π radians. Hence

[1-16]

Figure 1-4, Angular displacement of a simple pendulum (a) without irreversible energy and (b) with irreversible energy.

Suppose that the boundary that encloses the pendulum is not evacuated, but the pendulum oscillates in air or in some other viscous medium. If the pendulum is again started from an initial angle 00 the amplitudes of successive oscillations become smaller as shown in Fig. 1-4(b) and eventually the pendulum comes to a stop. The energy put into the system when the pendulum is displaced from θ = 0 to θ = θ₀ is converted into heat by the time the pendulum comes to a complete stop. Were it possible to insulate the boundary in which the pendulum is enclosed so that no heat could escape, all the energy put into the system would remain in the system. Equation 1-3 then could be modified to represent the energy relations as follows

[1-17]

The first term in brackets in the right-hand side of Eq. 1-17 is associated with the position of the pendulum and the second term with the velocity of the pendulum. By the time the pendulum ceases to oscillate, the gain in potential and kinetic energy has been converted into the gain in thermal energy. Equation 1-3 as applied to this case would be reduced to the following simple relationship

[1-18]

The thermal energy is irreversible since it cannot impart an angular displacement to the pendulum without some agency external to the system.

In practical situations, heat would escape from the system and the thermal energy would leave the system if the surrounding space were at a lower temperature. Whether or not the irreversible energy, or thermal energy in this case, leaves the system, it is necessary to maintain an energy flow into the system. This flow of energy must equal the irreversible energy in order to sustain the oscillations of the pendulum. In some types of clocks a main spring, which needs to be wound from time to time, is used to furnish the energy; in others a system of weights is used.