Volume-Temperature Measurements on Gases

Author: John Hutchinson

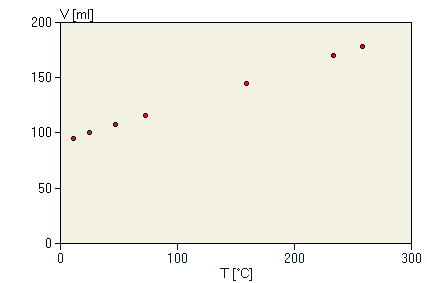
We have already noted the dependence of Boyle's Law on temperature. To observe a constant product of pressure and volume, the temperature must be held fixed. We next analyze what happens to the gas when the temperature is allowed to vary. An interesting first problem that might not have been expected is the question of how to measure temperature. In fact, for most purposes, we think of temperature only in the rather non-quantitative manner of "how hot or cold" something is, but then we measure temperature by examining the length of mercury in a tube, or by the electrical potential across a thermocouple in an electronic thermometer. We then briefly consider the complicated question of just what we are measuring when we measure the temperature.

Imagine that you are given a cup of water and asked to describe it as "hot" or "cold." Even without a calibrated thermometer, the experiment is simple: you put your finger in it. Only a qualitative question was asked, so there is no need for a quantitative measurement of "how hot" or "how cold." The experiment is only slightly more involved if you are given two cups of water and asked which one is hotter or colder. A simple solution is to put one finger in each cup and to directly compare the sensation. You still don't need a calibrated thermometer or even a temperature scale at all.

Finally, imagine that you are given a cup of water each day for a week at the same time and are asked to determine which day's cup contained the hottest or coldest water. Since you can no longer trust your sensory memory from day to day, you have no choice but to define a temperature scale. To do this, we make a physical measurement on the water by bringing it into contact with something else whose properties depend on the "hotness" of the water in some unspecified way. (For example, the volume of mercury in a glass tube expands when placed in hot water; certain strips of metal expand or contract when heated; some liquid crystals change color when heated; etc.) We assume that this property will have the same value when it is placed in contact with two objects which have the same "hotness" or temperature. Somewhat obliquely, this defines the temperature measurement.

For simplicity, we illustrate with a mercury-filled glass tube thermometer. We observe quite easily that when the tube is inserted in water we consider "hot," the volume of mercury is larger than when we insert the tube in water that we consider "cold." Therefore, the volume of mercury is a measure of how hot something is. Furthermore, we observe that, when two very different objects appear to have the same "hotness," they also give the same volume of mercury in the glass tube. This allows us to make quantitative comparisons of "hotness" or temperature based on the volume of mercury in a tube.

All that remains is to make up some numbers that define the scale for the temperature, and we can literally do this in any way that we please. This arbitrariness is what allows us to have two different, but perfectly acceptable, temperature scales, such as Fahrenheit and Centigrade. The latter scale simply assigns zero to be the temperature at which water freezes at atmospheric pressure. We then insert our mercury thermometer into freezing water, and mark the level of the mercury as "0". Another point on our scale assigns 100 to be the boiling point of water at atmospheric pressure. We insert our mercury thermometer into boiling water and mark the level of mercury as "100." Finally, we just mark off in increments of 1/100 of the distance between the "0" and the "100" marks, and we have a working thermometer. Given the arbitrariness of this way of measuring temperature, it would be remarkable to find a quantitative relationship between temperature and any other physical property.

Yet that is what we now observe. We take the same syringe used in the previous section and trap in it a small sample of air at room temperature and atmospheric pressure. (From our observations above, it should be clear that the type of gas we use is irrelevant.) The experiment consists of measuring the volume of the gas sample in the syringe as we vary the temperature of the gas sample. In each measurement, the pressure of the gas is held fixed by allowing the piston in the syringe to move freely against atmospheric pressure. A sample set of data is shown in table 3 and plotted here.

Sample Data from Volume-Temperature Measurement

Temperature (°C)	Volume (ml)
11	95.3
25	100.0
47	107.4
73	116.1
159	145.0
233	169.8
258	178.1

Figure 3: Volume vs. Temperature of a Gas.

We find that there is a simple linear (straight line) relationship between the volume of a gas and its temperature as measured by a mercury thermometer. We can express this in the form of an equation for a line:

V = αT+β

[5]

where V is the volume and T is the temperature in °C. α and β are the slope and intercept of the line, and in this case, α=0.335 and, β=91.7. We can rewrite this equation in a slightly different form:

V = α(T + β/α)

[6]

This is the same equation, except that it reveals that the quantity β/α must be a temperature, since we can add it to a temperature. This is a particularly important quantity: if we were to set the temperature of the gas equal to -(β/α) = -273°C, we would find that the volume of the gas would be exactly 0! (This assumes that this equation can be extrapolated to that temperature. This is quite an optimistic extrapolation, since we haven't made any measurements near to -273°C. In fact, our gas sample would condense to a liquid or solid before we ever reached that low temperature.)

Since the volume depends on the pressure and the amount of gas (Boyle's Law), then the values of a and β also depend on the pressure and amount of gas and carry no particular significance. However, when we repeat our observations for many values of the amount of gas and the fixed pressure, we find that the ratio -β/α = -273°C does not vary from one sample to the next. Although we do not know the physical significance of this temperature at this point, we can assert that it is a true constant, independent of any choice of the conditions of the experiment. We refer to this temperature as absolute zero, since a temperature below this value would be predicted to produce a negative gas volume. Evidently, then, we cannot expect to lower the temperature of any gas below this temperature.

This provides us an "absolute temperature scale" with a zero which is not arbitrarily defined. This we define by adding 273 (the value of β/α) to temperatures measured in °C, and we define this scale to be in units of degrees Kelvin (K). The data in table 3 are now recalibrated to the absolute temperature scale in table 4 and plotted here.

Analysis of Volume-Temperature Data

Temperature (°C)	Temperature (K)	Volume (ml)
11	284	95.3
25	298	100.0
47	320	107.4
73	350	116.1
159	432	145.0
233	506	169.8
258	531	178.1

Figure 4: Volume vs. Absolute Temperature of a Gas.

Note that the volume is proportional to the absolute temperature in degrees Kelvin,

V = kT

[7]

provided that the pressure and amount of gas are held constant. This result is known as Charles' Law, dating to 1787.

As with Boyle's Law, we must now note that the "constant" k is not really constant, since the volume also depends on the pressure and quantity of gas. Also as with Boyle's Law, we note that Charles' Law does not depend on the type of gas on which we make the measurements, but rather depends only the number of particles of gas. Therefore, we slightly rewrite Charles' Law to explicit indicate the dependence of k on the pressure and number of particles of gas

V = k(N,P)T

[8]