| Electronic Transformers and Circuits is a free introductory textbook on transformers and related circuits. See the editorial for more information.... |

|

Home  Amplifier Transformers Amplifier Transformers  Calculation of Inductance and Capacitance Calculation of Inductance and Capacitance |
||||||||||||||||||||||||






|
||||||||||||||||||||||||
Calculation of Inductance and Capacitance
where N = turns in windingFor concentric shell- or core-type windings the total leakage inductance referred to any winding is
where N = turns in that winding Winding capacitance is not expressible in terms of a single formula. The effective value of winding capacitance is almost never measurable, because it depends upon the voltages at the various points of the winding. The capacitance current at any point is equal to the voltage across the capacitance divided by the capacitive reactance. Since many capacitances occur at different voltages, in even the simplest transformer, no one general formula can suffice. The major components of capacitance are from 1. Turn to turn. 2. Layer to layer. 3. Winding to winding. 4. Windings to core. 5. Stray (including terminals, leads, and case). 6. External capacitors. 7. Vacuum-tube electrode capacitance. These components have different relative values in different types of windings. Turn-to-turn capacitance is seldom preponderant because the capacitances are in series when referred to the whole winding. Layer-to-layer capacitance may be the major portion in high-voltage single-section windings, where thick winding insulation keeps the winding-to-winding and winding-to-core components small. Items 5, 6, and 7 need to be watched carefully lest they spoil otherwise low-capacitance transformers and circuits. If a capacitance C with E1 volts across it is to be referred to some other voltage E2, the effective value at reference voltage E2 is
By use of equation 70 all capacitances in the transformer may be referred to the primary or secondary winding; the sum of these capacitances is then the transformer capacitance which is used in the various formulas and curves of preceding sections. In an element of winding across which voltage is substantially uniform throughout, capacitance to a surface beneath is
where A = area of winding element in square inches
If the winding element has uniformly varying voltage across it, as in Fig. 128, the effective capacitance is the sum of all the incremental effective capacitances. This summation is
where C = capacitance of winding element as found by equation 71 If E1 is zero and E2 = E, equation 72 becomes
or the capacitance, say, to ground of a single-layer winding with its low-voltage end grounded is one-third of the measured capacitance of the winding to ground. Measurement should be made with the winding ungrounded and both ends short-circuited together, to form one electrode, and ground to form the other. In a multilayer winding, E1 is zero at one end of each layer and E2 = 2E/NL at the other, where E is the winding voltage and NL is the number of layers. The effective layer-to-layer capacitance of the whole winding is
where CL is the measurable capacitance of one layer to another. The first and last layers have capacitance to other layers on one side only, and this is accounted for by the term in parentheses in equation 74.
Because the turns per layer and volts per layer are greater in windings with many turns of small wire, such windings have higher effective capacitance than windings with few turns. In a transformer with large turns ratio, whether step-up or step-down, this effective capacitance is often the barrier to further increase of turns ratio. With a given load impedance across the low impedance winding, there is a maximum effective capacitance Cm which can be tolerated for a given frequency response. If layer and winding capacitances have been reduced to the lowest practicable figure C1 the maximum turns ratio is Since effective capacitance is greater at higher voltages, in step-down transformers the capacitance may be regarded as existing mainly across the primary winding, in step-up transformers across the secondary winding. The effect of this on frequency response has been discussed in High-Frequency Response. The input capacitance of a triode amplifier is given by (1)
where CG-F = grid-to-cathode capacitance CG-F and CG-P are given for many tubes in the tube handbooks. They can be measured in any tube by means of a capacitance bridge.
|
||||||||||||||||||||||||
Home  Amplifier Transformers Amplifier Transformers  Calculation of Inductance and Capacitance Calculation of Inductance and Capacitance |
||||||||||||||||||||||||
Last Update: 2011-02-17



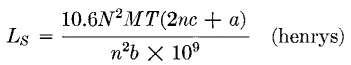






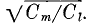 Appreciable amounts of capacitance across which large voltages exist must be eliminated by careful design.
Appreciable amounts of capacitance across which large voltages exist must be eliminated by careful design.
