Problems

In Problems 1-6, find the curl and divergence of the vector field.

7            Prove that for every vector field F(x, y, z) with continuous second partials, div(curl F) = 0.

8            Given a function f(x, y, z) with continuous second partials, show that

9            Use Stokes' Theorem to evaluate the surface integral where S is the portion of the paraboloid z = 1 - x² - y² above the (x, y) plane and F(x, y, z) = xy²i - x²yj + xyzk. (S is oriented with the top side positive.)

10            Use Stokes' Theorem to evaluate the line integral

where S is the portion of the plane z = 2x + 5y inside the cylinder x² + y² = 1 oriented with the top side positive.

11             Use Stokes' Theorem to evaluate the line integral

where S is the portion of the plane z = px + qy + r over a region D of area A, oriented with the top side positive.

12            Use Stokes' Theorem to show that the line integral

for any oriented surface S.

13            Use Gauss' Theorem to compute the surface integral

where E is the rectangular box 0 ≤ x ≤ a, 0 ≤ y ≤ b, 0 ≤ z ≤ c.

14            Use Gauss' Theorem to compute the surface integral

where E is the rectangular box 0 ≤ x ≤ 1l, 0 ≤ y ≤ 1, 0 ≤ z ≤ 1.

15            Use Gauss' Theorem to evaluate

where E is the region 0 ≤ x ≤ 1, 0 ≤ y ≤ x, 0 ≤ z ≤ x + y.

16            Use Gauss' Theorem to evaluate

where E is the sphere x² + y² + z² ≤ 4.

17            Use Gauss' Theorem to evaluate

where E is the hemisphere 0 ≤ z ≤ √(l - x² - y²).

18            Use Gauss' Theorem to evaluate

where S is the cylinder x² + y² ≤ 1, 0 ≤ z ≤ 4.

19            Use Gauss' Theorem to evaluate

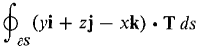
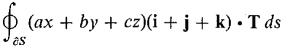
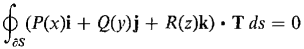
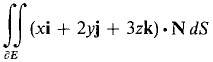
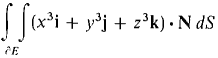
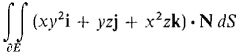
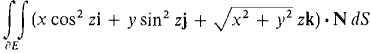
where E is the part of the cone above the (x, y) plane.