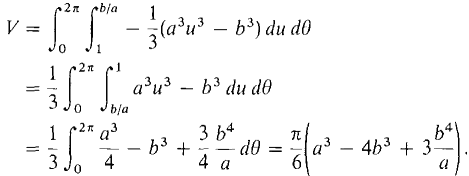The ebook Elementary Calculus is based on material originally written by H.J. Keisler. For more information please read the copyright pages.

Home Multiple Integrals Cylindrical and Spherical Coordinates Examples Example 4
 
del.icio.us Reddit Facebook StumbleUpon MySpace Twitter Search the VIAS Library  |  Index

Example 4

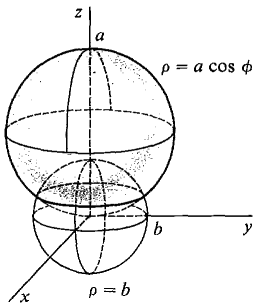
A sphere of diameter a passes through the center of a sphere of radius b, and a > b. Find the volume of the region inside the sphere of diameter a and outside the sphere of radius b.

Step 1

The region is sketched in Figure 12.7.19.

12_multiple_integrals-427.gif

Figure 12.7.19

Step 2

We let the z-axis be the line through the two centers and put the origin at the center of the sphere of radius b. The two spheres have the spherical equations

ρ = a cos φ, ρ = b.

They intersect at cos φ = b/a

Thus E is the region

0 ≤ θ ≤ 2π, 0 ≤ φ ≤ arccos (b/a) b ≤ ρ ≤ a cos φ.

Step 3

12_multiple_integrals-428.gif

Put u = cos φ, du = -sin φ dφ. Then

12_multiple_integrals-429.gif

Home Multiple Integrals Cylindrical and Spherical Coordinates Examples Example 4

Last Update: 2006-11-15