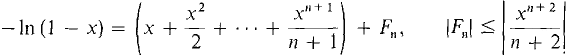Error Estimate

Our second method of approximation is to start with a known error estimate for the geometric series and carefully keep track of the error each time we make a new series.

We recall the formula for the partial sum of a geometric series.

Thus

This formula is valid for all x, but the error E_n approaches zero only when x is within the interval of convergence (-1, 1).

Example 4

Suppose we wish to approximate ln ½ within 0.01. If in the series

, r=l we set 1 - x = 2, x = ½, we get

We know this series converges, but to be sure of an approximation within 0.01 we need an error estimate. The next example shows how to get such an error estimate.

Example 5

Example 6

Example 7

Example 8

We know the series converges to something by the Alternating Series Test. For - 1 < x < 1 the series converges to ln (1 - x). But x = - 1 is an end-point of the interval of convergence and the general theorem on integrating a power series does not apply. So we must go back to the beginning and use the equation

For r ≤ 0, |tⁿ⁺¹/(1 - t)| ≤ |tⁿ⁺¹|. whence

= (1 + t + ... + tⁿ)+ E_n, |E_n[ ≤ |tⁿ⁺¹|. Integrating from 0 to x,

This holds for all x ≤ 0.

Now we set x = -1 and see that the error term |F_n| ≤ 1/(n + 2) approaches zero. This proves that ln 2 really is the sum of the alternating harmonic series,

ln 2 = 1 - ½ + ⅓ - ¼ + ....

The alternating harmonic series converges very slowly, because after n terms the error estimate is only 1/(n + 1).