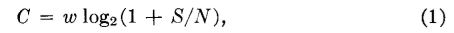Bandwidth and Noise in Communication Systems

With the development of pulse modulation, bandwidth requirements were reinvestigated by several independent groups^2,3 who found that the amount of interfering circuit noise in a system was also a factor determining bandwidth requirements. This has resulted in a modified law, which has been stated in several ways. Expressed mathematically,²

where C is the capacity of the channel to carry information per unit time, w is the bandwidth of the channel, and S/N is the signal-to-noise ratio in power units.

This law (which is sometimes referred to as the modified Hartley law) has very important implications. Thus, if the signal-to-noise power ratio is always maintained high, the bandwidth requirements may be low. Hence, in telephone, telegraph, or television transmission systems, if the power is always maintained high, less bandwidth is required by each signal for the same quality of transmission. Either more signals, or higher quality, are possible within a given bandwidth if the signal-to-noise power ratio is kept high.⁴

It has been pointed out² that the noise-reducing property of frequency modulation is in accordance with this principle. Thus, if an amplitude-modulated radio transmitter were modulated with an audio signal having a maximum frequency of 15000 cycles and if both sidebands were transmitted, the bandwidth required would be 30000 cycles. If a frequency-modulation radio transmitter of the broadcast type were modulated with the same signal, the bandwidth required would be about 200000 cycles wide. Hence, for comparable power conditions, a frequency-modulation station should give a considerable improvement in received signal-to-noise ratio. It appears that pulse-time modulation and particularly pulse-code modulation will be able to utilize the advantages indicated by this principle.⁴