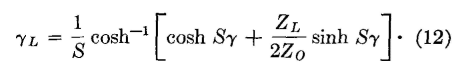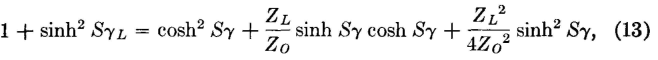Transmission Equations for Coil-Loaded Circuits

Figure 7. Method of inserting loading coils in a cable pair. The coils at each loading point are inductively coupled.

It is seen from Fig. 8 that the characteristics of the final loaded cable are a combination of the section A-B, C-D having distributed constants and the lumped loading coils Z_L/2 at each end. The propagation constant γ_L and the characteristic impedance Z_0L of the final

Figure 8. Network equivalent to Fig. 7.

Figure 9. The T section of a uniform line that is equivalent to the loaded circuit of Figs. 7 and 8.

loaded cable may be considered the propagation constant and the characteristic impedance of an exactly equivalent uniform cable represented diagrammatically by the T section of Fig. 9.

Adding the loading coils to the circuit does not appreciably change the shunt paths of Figs. 8 and 9. It can therefore be written that

Again referring to these figures, it is seen that

Then,

When the value of Z_OL given by equation 8 is substituted in equation 10,

and

This is the Campbell formula⁵ for a loaded cable, giving the propagation constant γ_L of the loaded cable in terms of the values of the unloaded cable and the impedance of the loading coils. Considerable mathematical reduction is required between equations 10 and 11; this will be found on page 181 of reference 6.

As shown in reference 6, equation 11 can be written (since cosh² x = sinh²x + 1),

and this can be reduced to the form

When equation 14 is substituted in equation 8, it follows that

This formula gives the mid-load or mid-coil characteristic impedance of the lump-loaded cable in terms of the constants of the cable, before loading, and the impedance of the loading coils. A similar equation can be derived for the midsection termination.