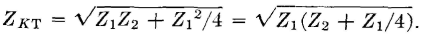Transmitted Band from Iterative Impedances

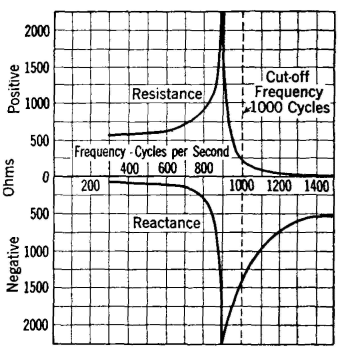
Now suppose that a frequency is chosen such that the iterative impedance of the filter is purely resistive. At this frequency, the source will send power to the filter, and, since the filter cannot absorb power, it must reach the load.

Next assume that at a different frequency the iterative impedance of the filter is purely reactive. This prevents the source from sending any power into the connected circuit, and thus no power reaches the load at this frequency. From these facts it may be concluded that a filter will transmit without attenuation those frequencies for which the iterative impedance Z_K is resistive (Fig. 25).

The iterative impedance given by equation 45 is

Figure 25. Approximate resistance and reactance components of the iterative impedance of a typical low-pass filter.

For unattenuated transmission this must be a positive real number to be resistive. This equation consists of two parts: X_a = Z₁ and X_b = (Z₂+Z₁/4). Thus, Z_KT = sqrt(X_aX_b). Pure reactances only are being considered; and for Z_KT to be positive, if X_a represents inductive reactance and is +jN_a, then X_b must be capacitive reactance or -jX_b, and vice versa, within the band of frequencies transmitted. For these relations Z_KT = sqrt((+jX_a)(-jX_b)), and Z_KT will be positive. To satisfy these conditions, Z₂ must be, first, the opposite type of reactance to Z₁, and second, greater in numerical value than Z₁/4. Or, in other words, the ratio Z₁/4Z₂ from equation 45 must lie between 0 and -1 for the frequencies to be transmitted, as shown in Fig. 26.